Mathieu Ossendrijver is an astroarchaeologist at Humboldt Universit...
The planet Jupiter has been known since ancient times. It is visibl...
On four of these tablets, the distance covered by Jupiter is comput...
The mean speed theorem, also known as the Merton rule of uniform ac...
Jupiter moves across the sky in a very predictable pattern, but eve...
Knowing that ancient Babylonians had access to this geometrical met...
The use of a graph to understand the motion or speed over time has ...
eastern tropical Pacific and Antarctica peaked
during each of the last two glacial terminations
(28), consistent with the timing of enhanced EPR
hydrothermal activity.
Isolating a mechanistic linkage between ridge
magmatism and glacial terminations will require
a suite of detailed proxy records from multiple
ridges that are sensitive to mantle carbon and
geothermal inputs, as well as modeling studies
of their influence in the ocean interior. The
EPR results establish the timing of hydrothermal
anomalies, an essential prerequisite for deter-
mining whether ridge magmatism can act as a
negative feedback on ice-sheet size. The data
presented here demonstrate that EPR hydro-
thermal output increased after the two largest
glacial maxima of the past 200,000 years, im-
plicating mid-ocean ridge magmatism in glacial
terminations.
REFERENCES AND NOTES
1. D. C. Lund, P. D. Asimow, Geochem. Geophys. Geosyst. 12,
Q12009 (2011).
2. P. Huybers, C. Langmuir, Earth Planet. Sci. Lett. 286, 479–491
(2009).
3. J. W. Crowley, R. F. Katz, P. Huybers, C. H. Langmuir,
S. H. Park, Science 347, 1237–1240 (2015).
4. M. Tolstoy, Geophys. Res. Lett. 42, 1346–1351 (2015).
5. E. T. Baker, G. R. German, in Mid-Ocean Ridges: Hydrothermal
Interactions Between the Lithosphere and Oceans,
C. R. German, J. Lin, L. M. Parson, Eds. (Geophysical
Monograph Series vol. 148, American Geophysical Union,
2004), pp. 245–266.
6. S. E. Beaulieu, E. T. Baker, C. R. German, A. Maffei, Geochem.
Geophys. Geosyst. 14, 4892–4905 (2013).
7. E. T. Baker, Geochem. Geophys. Geosyst. 10, Q06009 (2009).
8. J. Dymond, Geol. Soc. Am. 154, 133–174 (1981).
9. G. B. Shimmield, N. B. Price, Geochim. Cosmochim. Acta 52,
669–677 (1988).
10. K. G. Speer, M. E. Maltrud, A. M. Thurnherr, in Energy and Mass
Transfer in Hydrothermal Systems, P. E. Halbach, V. Tunnicliffe,
J. R. Hein, Eds. (Dahlem University Press, 2003), pp. 287–302.
11. K. Boström, M. N. Peterson, O. Joensuu, D. E. Fisher, J. Geophys.
Res. 74,3261–3270 (1969).
12. M. Frank et al., Paleoceanography 9,559–578
(1994).
13. Material and methods are available as supplemental materials
on Science Online.
14. C. R. German, S. Colley, M. R. Palmer, A. Khripounoff,
G. P. Klinkhammer, Deep Sea Res. Part I Oceanogr. Res. Pap.
49, 1921–1940 (2002).
15. R. R. Cave, C. R. German, J. Thomson, R. W. Nesbitt, Geochim.
Cosmochim. Acta 66, 1905–1923 (2002).
16. T. Schaller, J. Morford, S. R. Emerson, R. A. Feely, Geochim.
Cosmochim. Acta 64, 2243–2254 (2000).
17. S. Emerson, J. I. Hedges, in Treatise on Geochemistry,
K. K. Turekian, H. D. Holland, Eds. (Elsevier, vol. 6, 2004),
pp. 293–319.
18. J. A. Goff, Science 349, 1065 (2015).
19. J. A. Olive et al., Science 350, 310–313 (2015).
20. P. U. Clark et al., Science 325, 710–714 (2009).
21. K. Key, S. Constable, L. Liu, A. Pommier, Nature 495, 499–502
(2013).
22. P. B. Kelemen, G. Hirth, N. Shimizu, M. Spiegelman, H. J. B. Dick,
Philos. Trans. R. Soc. Lond. A 355,283–318 (1997).
23. J. Maclennan, M. Jull, D. McKenzie, L. Slater, K. Gronvold,
Geochem. Geophys. Geosyst. 3,1–25 (2002).
24. P. Cartigny, F. Pineau, C. Aubaud, M. Javoy, Earth Planet.
Sci. Lett. 265, 672–685 (2008).
25. J. M. A. Burley, R. F. Katz, Earth Planet. Sci. Lett. 426, 246–258
(2015).
26. M. Hofmann, M. A. Morales Maqueda, Geophys. Res. Lett. 36,
L03603 (2009).
27. J. Emile-Geay, G. Madec, Ocean Sci. 5, 203–217 (2009).
28. P. Martin, D. Archer, D. W. Lea, Paleoceanography 20, PA2015
(2005).
29. D. K. Smith, H. Schouten, L. Montési, W. Zhu, Earth Planet.
Sci. Lett. 371–372,6–15 (2013).
30. L. E. Lisiecki, M. E. Raymo, Paleoceanography 20, PA1003
(2005).
ACK NOW LE DGM EN TS
We dedicate this paper to J. Dymond, whose 1981 treatise on Nazca
plate sediments made this work possible. We are also indebted
to the Oregon State University Core Repository for carefully
preserving the EPR sediment cores since they were collected in
the early 1970s. We are grateful to L. Wingate at the University of
Michigan and M. Cote at the University of Connecticut for
technical support. This work has benefited from discussions
with J. Granger, P. Vlahos, B. Fitzgerald, and M. Lyle. Data
presented here are available on the National Oceanic and
Atmospheric Administration’s Paleoclimatology Data website
(www.ncdc.noaa.gov/data-access/paleoclimatology-data). Funding
was provided by the University of Michigan and the University
of Connecticut.
SUPPLEMENTARY MATERIALS
www.sciencemag.org/content/351/6272/478/suppl/DC1
Materials and Methods
Supplementary Text
Figs. S1 to S11
Tables S1 to S5
References (31–45)
14 September 2015; accepted 6 January 2016
10.1126/science.aad4296
HISTORY OF SCIENCE
Ancient Babylonian astronomers
calculated Jupiter’s position from the
area under a time-velocity graph
Mathieu Ossendrijver*
Theideaofcomputingabody’s displacement as an area in time-velocity space is usually traced
back to 14th -century Europe. I show that in four ancient Babylonian cuneiform tablets, Jupiter’s
displacement along the ecliptic is computed as the area of a trapez oidal figure obtained by
drawing its daily displacement ag ainst time. This interpretation is prompted by a newly
discov er ed tablet on which the same computation is presented in an equivalent arithmetical
formulation. The tablets date from 350 to 50 BCE. The trape z oid procedures offer the first
evidence for the use of geometrical methods in Bab ylonian mathematical astronomy , which was
thus far viewed as operating e xclusively with arithmetical concepts.
T
he so-called trapezoid procedures examined
in this paper have long puzzled historians
of Babylonian astronomy. They belong to
the corpus of Babylonian mathematical as-
tronomy, which comprises about 450 tab-
lets from Babylon and Uruk dating between 400
and 50 BCE. Approximately 340 of these tablets
are tables with computed planetary or lunar data
arranged in rows and columns (1). The remaining
110 tablets are proced ure texts with computa-
tional instructions (2), mostly aimed at comput-
ing or verifying the tables. In all of these texts the
zodiac, invented in Babylonia near the end of the
fifth century BCE (3), is used as a coordinate sys-
tem for computing celestial positions. The un-
derlying algorithms are structured as branching
chains of arithmetical operations (additions, sub-
tractions, and multiplications) that can be rep-
resented as flow charts (2). Geometrical concepts
are conspicuously absent from these texts, whereas
they are very common in the Babylonian mathe-
matical corpus (4–7). Currently four tablets, most
likely written in Babylon between 350 and 50 BCE,
are known to preserve portions of a trapezoid
procedure (8). Of the four procedures, here labeled
B to E (figs. S1 to S4), one (B) preserves a men-
tion of Jupiter and three (B, C, E) are embedded
in compendia of procedures dealing exclusively
with Jupiter. The previously unpublished text D
probably belongs to a similar compendium for
Jupiter. In spite of these indications of a connec-
tion with Jupiter, their astronomical significance
was previously not acknowledged or understood
(1, 2, 6).
A recently discovered tablet containing an un-
published procedure text, here labeled text A (Fig. 1),
sheds new light on the trapezoid procedures. Text A
most likely originates from the same period and
location (Babylon) as texts B to E (8). It contains
a nearly complete set of instructions for Jupiter’s
motion along the ecliptic in accordance with the
so-called scheme X.S
1
(2). Before the discovery of
text A, this scheme was too fragmentarily known
for identifying its connection with the trapezoid
procedures. Covering one complete synodic cycle,
scheme X.S
1
begins with Jupiter’s heliacal rising
(first visible rising at dawn), continuing with its
first station (beginning of appa rent retrograde
motion), acronychal rising (last visible rising at
dusk), second station (end of retrograde motion),
and heliacal setting (last visible setting at dusk)
(2). Scheme X.S
1
and the four trapezoid procedures
are here shown to contain or imply mathematically
equivalent descriptions of Jupiter’smotionduring
the first 60 days after its first appearance. Whereas
scheme X.S
1
employs a purely arithmetical ter-
minology, the trapezoid procedures operate with
geometrical entities.
482 29 JANUARY 20 16 • VOL 351 ISSUE 6272 sciencemag.org SCIENCE
Excellence Cluster TOPOI–Institute of Philosophy, Humboldt
University, Berlin, Germany.
*Corresponding author. E-mail: mathieu.ossendrijver@hu-berlin.de
RESEARCH | REPORTS
In text A, Jupiter’s motion along the ecliptic is
described in terms of its daily displacement (mod-
ern symbol: v) expressed in °/d (degrees/day) and
its total displacement (S) expressed in degrees. A
crucial new insight about scheme X.S
1
provided
bytextAconcernsitsuseofpiecewiselinearly
changing values for v. Although not formulated
explicitly, this linear dependence on time is clearly
implied (8). Jupiter’smotionalongtheeclipticis
described for two consecutive intervals of 60 days
between its first appearance and its first station.
For each interval, initial and final values of v are
provided. Note that Babylonian astronomy em-
ploys a sexagesimal; i.e., base-60 place-value system
in which numbers are represented as sequences of
digits between 0 and 59, each associated with a
power of 60 that decreases in the right direction. In
the commonly used modern notation for these
numbers, all digits are separated by commas, ex-
cept for the digit pertaining to 60°, which is
separated from the next one pertaining to 60
−1
by a semicolon (;), the analog of our decimal point.
For the first interval of 60 days, v
0
= 0;12°/d (=12/60)
and v
60
= 0;9,30°/d (=9/60 + 30/60
2
). Their sum
is multiplied by 0;30 (=1/2), resulting in a mean
value (v
0
+ v
60
)/2 = 0;10,45°/d, which is multi-
plied by 1,0 (=60) days, resulting in a total
displacement S =1,0•(v
0
+ v
60
)/2 = 10;45°. For
the second interval, v
60
= 0;9,30°/d and v
120
=
0;1,30°/d (=1/60 + 30/60
2
), leading to (v
60
+
v
120
)/2 = 0;5,30°/d and S = 5;30°. The sum of
the total displacements, 10;45° + 5;30° = 16;15°, is
declared to be the total distance by which Jupiter
proceeds along the ecliptic in 120 days. In other
words, the ecliptic longitude of Jupiter after 60
and 120 days is computed as l
60
= l
0
+10;45°
and l
120
= l
0
+ 16;15°, respectively.
Text A does not describe how v varies from day
to day, but of the three forms of time dependence
of v that are attested in Babylonian planetary
texts—piecewise constant, linear , or quadratic in
each time interval (2, 9)—only the linear one comes
into question. If v were piecewise constant, then
S should equal 60•v for each interval. If v were
piecewise quadratic, then S =60•(v
0
+ v
60
)/2 can
only be some rough approximation. That would
be unexpected, since other tablets imply that some
Babylonian scholars in this period were familiar
with the exact algorithm for summing a quadratic
series (9, 10). By contrast, the values of S computed
in text A are exact if one assumes that v changes
linearly in each interval. It follows that in scheme
X.S
1
, v decreases linearly from 0;12°/d to 0;9,30°/d
between day 0 and day 60, and from 0;9,30°/d to
0;1,30°/d between day 60 and day 120.
This new reconstruction of the first 120 days of
scheme X.S
1
results in trapezoidal figures if v is
plotted against time in a modern fashion (Fig. 2).
It is important to note that text A itself does not
contain or imply a geometrical representation.
However, it turns out to be explicitly formulated
in the trapezoid procedures, texts B to E (figs. S1
to S4). Although their formulation differs in details,
at least three of them (B to D) consist of the same
two parts, I and II.
In part I, Jupiter’stotaldisplacementforthe
first 60 days of scheme X.S
1
is computed. A cor-
responding introductory statement mentioning
Jupiter and the measures of the trapezoid is part-
ly preserved in texts B and C, and perhaps in text
E(8). The number 10;45, referred to as th e “area”
of the trapezoid (B, C), is then added to the “po-
sition of appearance” (B, C, D), the technical term
for Jupiter’s ecliptical longitude at first appearance,
i.e., l
60
= l
0
+ 10;45°. Texts B and C partly preserve
the computation of 10;45 as the area of the trap-
ezoid through a series of steps equivalent to the
computations in text A. Its “large side” and “small
side,” v
0
=0;12°/dandv
60
=0;9,30°/d,areav-
eraged, (v
0
+ v
60
)/2 = 0;10,45°/d, which is then
multiplied by 60 days, the width of the trapezoid,
resulting in 10;45°. The latter operation is partly
preserved in text C and can be restored in text B.
Part II, partly preserved in texts B, D, and E, is
concerned with the time in which Jupiter reaches
a position referred to by a term tentatively trans-
lated as the “crossing” (8). It is now clear that this
denotes a point on the ecliptic, say l
c
, located
halfway between l
0
and l
60
, i.e., l
c
= l
0
+10;45°/2.
This interpretation is consistent with a statement,
preserved only in text B, according to which the
“crossing” is located in the middle of Jupiter’s
“path,” readily interpreted as a reference to the
ecliptical segment from l
0
to l
60
.TextsBand
D also preser ve the following sta tement that
SCIENCE sciencemag.org 29 JA NUARY 2016 • VOL 351 ISSUE 6272 483
Fig. 1. Photograph of text A (lines 1 to 7). (A) Full image. (B) Partial image of the right side taken
under different lighting conditions.
0,20,1
0;12
0;10
0;8
0;6
0;4
0;2
0
first station
0;1,30
0;5,30
0;9,30
0;10,45
0
first appearance
time [days]
v [
o
/day]
5;30°10;45°
Fig. 2. Time-velocity graph of Jupiter’smotion.Daily displacement along the ecliptic (v) between
Jupiter’s first appearance (day 0) and its first station (day 120) as a function of time according to scheme X.S
1
as inferred from text A. All numbers and axis labels are in sex agesi mal place-value notation.The areas of the
trapez oids, 10;45° and 5;30°, each represent Jupiter’s total displacement during one interval of 60 days.
RESEARCH | REPORTS
precedes the solution procedure: “ Concerning
this 10;45, you see when it is halved.” The time
in which Jupiter reaches l
c
,sayt
c
,isthencom-
puted by the following geometrical method: The
trapezoid for days 0 to 60 is divided into two
smaller trapezoids of equal area (Fig. 3). In order
toachievethis,theBabylonianastronomersap-
plied a partition procedure that is well-attested in
Old Babylonian (2000 to 1800 BCE) mathematics
(5, 6). In modern terms, it can be formulated as
follows: If v
0
and v
60
are the parallel sides of a
trapezoid, then the intermediate parallel that
divides it into two trapezoids of equal area has a
height v
c
=[(v
0
2
+ v
60
2
)/2]
1/2
. In the present case,
v
c
denotes Jupiter’s daily displacement when it
is at the “crossing.” This expression follows from
equating the areas of the partial trapezoids, S
1
=
t
c
•(v
0
+ v
c
)/2 = S
2
= t
2
•(v
c
+ v
60
)/2, where t
c
and t
2
are the widths of these trapezoids, and using t
c
=
t•(v
0
– v
c
)/(v
0
– v
60
), where t=t
c
+t
2
is the
width of the original trapezoid (6, 10). Inserting
v
0
=0;12°/d,v
60
=0;9,30°/d,andt =1,0d,weob-
tain v
c
=[(0;2,24+0;1,30,15)/2]
1/2
= (0;1,57,7,30)
1/2
=
0;10,49,20,44,58,...°/d, t
c
= 28;15,42,0,48,...d, and
t
2
= 31;44,17,59,12,...d. The computation of v
c
is
partly preserved in text D up to the addition
0;2,24 + 0;1,30,15 (8). In text B, the related quan-
tity u
2
=(v
0
2
– v
60
2
)/2 = (0;2,24 – 0;1,30,15)/2 =
0;0,26,52,30 is computed. This was most likely
followed by another step in which v
c
was com-
puted using v
c
2
= v
0
2
– u
2
. Whereas all known
Old Babylonian examples of the partition algo-
rithm concern trapezoids for which v
c
, v
0
,and
v
60
are terminating sexagesimal numbers (6), the
present solution does not terminate in the sex-
agesimal system. Hence, texts B to E can only
have offered rounded results for v
c
and t
c
.Nothing
remains of this in texts B to D, but text E partly
preserves a computation involving 0;10,50, which
is, most plausibly, an approximation of v
c
.This
interpretation is confirmed by the fact that text
E also mentions the value t
c
= 28 d and, very
likely, t
2
= 32 d, both in exact agreement with
t
c
=60•(v
0
– v
c
)/(v
0
– v
60
) and t
2
=60– t
c
if one
approximates v
c
= 0;10,50°/d. By rounding v
c
,
only an approximately equal partition of the trap-
ezoid is achieved.
Also partly preserved in text E is a computa-
tion of the area of the second partial trapezoid,
using the same method as before, leading to S
2
=
t
2
•(v
c
+ v
60
)/2, whe re t
2
=32days,v
c
=0;10,50°/d,
and v
60
=0;9,30°/d.ThevalueofS
2
is broken
away but can be restored as 5;25,20°. The probable
purpose of this computation was to verify the
solution for v
c
,asisdoneintheOldBabylonian
mathematical text UET 5, 858 (5, 11). The anal-
ogous computation of the area of the first par-
tial trapezoid, which can be reconstructed as S
1
=
t
c
•(v
0
+ v
c
)/2 = 5;19,40°, is not preserved. Neither
of these values equals 5;22,30° = S/2 as they
ideally should (Fig. 3), a direct consequence of the
rounding of v
c
to 0;10,50°/d. At most two more
lines are partl y preserved in texts B, D, and E, but
they are too fragmentary for an interpretation.
The evidence presented here demonstrates
that Babylonian astronomers construed Jupiter’s
displacement along the ecliptic during the first
60 days after its first appearance as the area of a
trapezoid in time-velocity space. Moreover, they
computed the time when Jupiter covers half this
distance by partitioning the trapezoid into two
smaller ones of ideally equal area. These compu-
tations predate the use of similar techniques by
medieval European scholars by at least 14 cen-
turies. The “Oxford calculators” of the 14th cen-
tury CE, who were centered at Merton College,
Oxford, are credited with formulating the “Mer-
tonian mean speed theorem” for the distance
traveled by a uniformly accelerating body, cor-
responding to the modern formula s = t•(v
0
+
v
1
)/2, where v
0
and v
1
are the initial and final
velocities (12, 13). In the same century Nicole
Oresme, in Paris, devised graphical methods that
enabled him to prove this relation by computing
s as the area of a trapezoid of width t and heights
v
0
and v
1
(12). Part I of the Babylonian trapezoid
procedures can be viewed as a concrete example
of the same computation. They also show that
Babylonian astronomers did, at least occasionally,
use geometrical methods for computing planetary
positions. Ancient Greek astronomers such as
Aristarchus of Samos, Hipparchus, and Claudius
Ptolemy also used geometrical methods ( 12),
while arithmetical methods are attested in the
Antikythe ra mechanism (14)andinGreco-Roman
astronomical papyri from Egypt (15). However ,
the Babylonian trapezoid procedures are geo-
metrical in a different sense than the methods
of the mentioned Greek astronomers, since the
geometrical figures describe configurations not
in physical space but in an abstract mathemat-
ical space defined by time and velocity (daily
displacement).
REFERENCES AND NOTES
1. O. Neugebauer, Astronomical Cuneiform Texts (Lund Humphries,
London, 1955).
2. M. Ossendrijver, Babylonian Mathematical Astronomy:
Procedure Texts (Springer, New York, 2012).
3. J. P. Britto n, Arch. Hist. Exact Sci. 64,617–663
(2010).
4. J. Høyr up, Lengths, Widths, Surfaces. A Portrait of Old
Babylonian Algebra and Its Kin (Springer, New York,
2002).
5. A. A. Vaiman, Shumero-Vavilonskaya matematika III-I
tysyacheletiya do n. e. (Izdatel’stvo Vostochnoy Literatury,
Moscow, 1961).
6. J. Friberg, in Reallexikon der Assyriologie, D. O. Edzard, Ed.
(De Gruyter, Berlin, 1990), vol. 7, pp. 561–563.
7. J. Friberg, A Remarkable Collection of Babylonian Mathematical
Texts. Manuscripts in the Schøyen Collection: Cuneiform
Texts I (Springer, New York, 2007).
8. Materials and methods are available as supplementary
materials on Science Online.
9. P. J. Huber, Z. Assyriol. 52, 265–303 (1957).
10. O. Neugebauer, Mathematische Keilschrifttexte, I–III (Springer,
Berlin, 1935–1937).
11. J. Friberg, Rev. Assyriol. Archeol. Orient. 94,97–188
(2000).
12. O. Pedersen, Early Physics and Astronomy. A Historical
Introduction (Cambridge Univ. Press, Cambridge, 1974).
13. E. D. Sylla, in The Cambridge History of Later Medieval
Philosophy, N. Kretzmann, A. Kenny, J. Pinborg, Eds.
(Cambridge Univ. Press, Cambridge, 1982), pp. 540–563.
14. T. Freeth, A. Jones, J. M. Steele, Y. Bitsakis, Nature 454,
614–617 (2008).
15. A. Jones, Astronomical Papyri from Oxyrhynchus (American
Philosophical Society, Philadelphia, 1999).
AC KN OW LE D GM E NT S
The Trustees of the British Museum (London) are thanked for
permission to photograph, study, and publish the tablets. Work
was supported by the Excellence Cluster TOPOI, “The Formation
and Transformation of Space and Knowledge in Ancient Cultures”
(Deutsche Forschungsgemeinschaft grant EXC 264), Berlin.
Photographs, transliterations, and translations of the relevant parts
of the tablets are included in the supplementary materials. The
tablets are accessible in the Middle Eastern Department of the
British Museum under the registration numbers BM 40054
(text A), BM 36801, BM 41043, BM 34757 (text B), BM
34081+34622+34846+42816+45851+46135 (text C), BM 35915
(text D), and BM 82824+99697+99742 (text E). H. Hunger (Vienna)
is acknowledged for providing an unpublished photograph of
BM 40054.
SUPPLEMENTARY MATERIALS
www.sciencemag.org/content/351/6272/482/suppl/DC1
Materials and Methods
Figs. S1 to S4
References (16–21)
4 November 2015; accepted 23 December 2015
10.1126/science.aad8085
484 29 JANUARY 2016 • VOL 351 ISSUE 6272 sciencemag.org SCIE NCE
Fig. 3. Partitioning the trapezoid for
days 0 to 60. The time at which
Jupiter reaches the “crossing,” t
c
,
where it has covered the distance
5;22,30° = 10;45°/2, is computed
geometrically by dividing the trapezoid
for days 0 to 60 into two smaller
trapez oids of equal area. In text E, v
c
is
rounded to 0;10,50°/d, resulting in t
c
=
28 d, S
1
=5;19,40°,t
2
=32d,andS
2
=
5;25,20°.
0;12
0;10
0;8
0;6
0;4
0;2
=0;12
=0;10,49,20,...
=0;9,30v
v
v
0
0 =28;15,42,... 1,0t
oo
0
60
c
c
time [days]
v [
o
/day]
5;22,30 5;22,30
RESEARCH | REPORTS
The mean speed theorem, also known as the Merton rule of uniform acceleration, was first discovered in the 14th century by the Oxford Calculators of Merton College, and was proved by Nicole Oresme. It states that a uniformly accelerated body (starting from rest, i.e. zero initial velocity) travels the same distance as a body with uniform speed whose speed is half the final velocity of the accelerated body.
This new clay tablets prove that Babylonian astronomers antecipated the theorem by 1400 years!
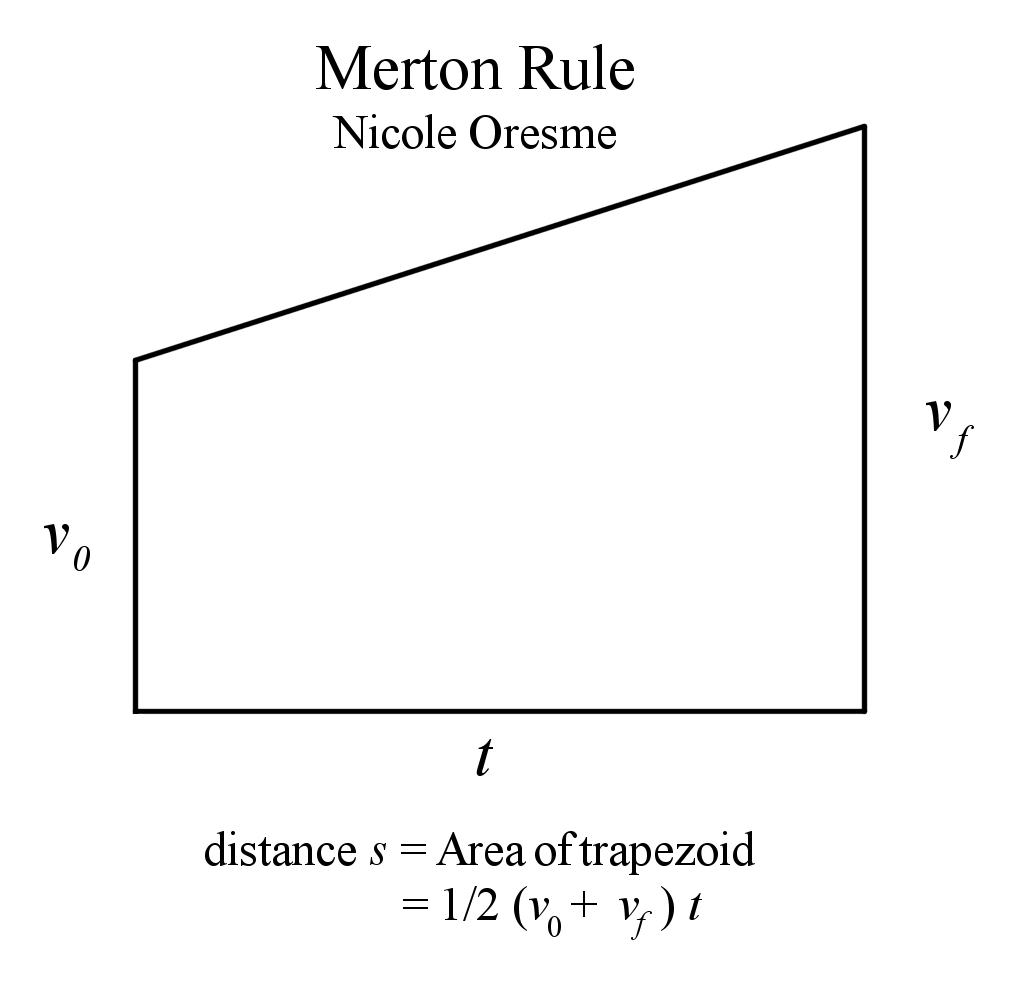
Knowing that ancient Babylonians had access to this geometrical methods provides a whole new context for examining previously discovered tablets, as many tablets that are already translated have sections that aren't yet understood.
On four of these tablets, the distance covered by Jupiter is computed as the area of a figure that represents how its velocity changes with time. None of the tablets contains drawings but, as Mathieu Ossendrijver explains, the texts describe the figure of which the area is computed as a trapezoid. Two of these so-called trapezoid texts had been known since 1955, but their meaning remained unclear, even after two further tablets with these operations were discovered in recent years.
One reason for this was the damaged state of the tablets, which were excavated unscientifically in Babylon, near its main temple Esagila, in the 19th century. Another reason was, that the calculations could not be connected to a particular planet. The new interpretation of the trapezoid texts was now prompted by a newly discovered, almost completely preserved fifth tablet. A colleague from Vienna who visited the Excellence Cluster TOPOI in 2014, the retired Professor of Assyriology Hermann Hunger, draw the attention of Mathieu Ossendrijver to this tablet. He presented him with an old photograph of the tablet that was made in the British Museum.
The new tablet does not mention a trapezoid figure, but it does contain a computation that is mathematically equivalent to the other ones. This computations can be uniquely assigned to the planet Jupiter.
Mathieu Ossendrijver is an astroarchaeologist at Humboldt University of Berlin. Ossendrijver was an astrophysicist before he began studying the history of science and cuneiform in 2005. In 2012, he published a book with new translations of the known Babylonian tablets that featured astronomical calculations and several new tables.

Jupiter moves across the sky in a very predictable pattern, but every now and then it reverses direction in the sky, making a tiny loop against the background stars – this is Jupiter retrograde.

Here's a [video](https://www.youtube.com/watch?v=cmA9ucnsBBw) of Jupiter's retrograde motion in 2010.
The planet Jupiter has been known since ancient times. It is visible to the naked eye in the night sky and can occasionally be seen in the daytime when the Sun is low. To the Babylonians, this object represented their god Marduk. They used Jupiter's roughly 12-year orbit along the ecliptic to define the constellations of their zodiac.
The use of a graph to understand the motion or speed over time has been usually traced back to scholars in Oxford and Paris around 1350, and then to Isaac Newton, but these new discoveries are a testament of the brilliance of the unknown Mesopotamian scholars who constructed Babylonian mathematical astronomy.