### TL;DR
This is a text about differentiation and its geometric...
You can find the entire book here:
- [Calculus Made Easy (HTML v...
Silvanus Phillips Thompson was a professor of physics mostly known ...
A visual example of varying slopes at different points of a curve. ...
The tangent to the curve at specific point is parametrized by a lin...
That's funny
Values for the tangent function $\tan$ between $-2\pi$ and $2\pi$. ...
ahhhh
local minimum
In this figure we can see one maximum at $x=1$ and one minimum at $...
The simplest case to examine is that of a linear function. The slop...
This is a great example where you can visualize the graph for a giv...
let's talk about this
CHAPTER X.
GEOMETRICAL MEANING OF DIFFERENTIATION.
It is useful to consider what geometrical meaning can be given to the
di↵erential coefficient.
In the first place, any function of x,such,forexample,asx
2
,or
p
x,
or ax + b,canbeplottedasacurve;andnowadayseveryschoolboyis
familiar with the process of curve-plotting.
dx
dy
P
Q
R
y
x
dx
O X
Y
Fig. 7.
Let PQR,inFig. 7,beaportionofacurveplottedwithrespect
to the axes of coordinates OX and OY .ConsideranypointQ on this
curve, where the abscissa of the point is x and its ordinate is y. Now
observe how y changes w hen x is varied. If x is made to incr ea se by
CALCULUS MADE EASY 76
asmallincrementdx,totheright,itwillbeobservedthaty also (in
this particular curve) increases by a small increment dy (because this
particular curve happens to be an ascending curve). Then the ratio of
dy to dx is a measure of the degree to which the curve is sloping up
between the two points Q and T . As a matter of fact, it can be seen on
the figure that the curve between Q and T has m a ny di↵erent slopes,
so that we cann ot very well speak of the slope of the curve between
Q and T .If,however,Q and T are so near each other that the small
portion QT of the curve is practically straight, then it is true to say that
the ratio
dy
dx
is the slope of the curve along QT .ThestraightlineQT
produced on either side touches the curve along the portion QT only,
and if this portio n is indefinitely small, the straight line will touch the
curve at practically one point only, and be therefore a tangent to the
curve.
This tangent to th e curve has evidently the same slope as QT ,so
that
dy
dx
is the slope of the tangent to the curve at the point Q for which
the value of
dy
dx
is found.
We have seen that the short expression “the slope of a curve” has
no precise meaning, because a curve has so many slopes—in fact, every
small portion of a curve has a di↵erent slope. “The slope of a curve at
a point”is,however,aperfectlydefinedthing;itistheslopeofavery
small portion of the curve situated just at that point; and we have seen
that this is the same as “the slope of the tan gent to the curve at that
point.”
Observe that dx is a short step to the right, and dy the correspond-
ing short step upwards. These steps must be considered as short as
MEANING OF DIFF ER ENTI ATION 77
possible—in fact indefinitely short,—though in diagrams we have to
represent them by bits that are not infini t es i mal l y small, otherwise
they could not be seen.
We shall hereafter make considerable use of this circumstance that
dy
dx
represents the slope of the curve at any point.
dx
dy
O X
Y
Fig. 8.
If a curve is sloping up at 45
at a particular point, as in Fig. 8, dy
and dx will be equal, and the value of
dy
dx
=1.
If the curve slopes up steeper t h an 45
(Fig. 9),
dy
dx
will be greater
than 1.
If the curve slopes up very gently, as i n Fig. 10,
dy
dx
will be a fraction
smaller than 1.
For a horizontal line, or a horizontal place in a curve, dy =0,and
therefore
dy
dx
=0.
If a curve slopes downward,asinFig. 11, dy will be a step down,
and must t h er efo re be reckoned of negative va l u e; hence
dy
dx
will have
negative sign also.
CALCULUS MADE EASY 78
dx
dy
O X
Y
Fig. 9.
dx
dy
O X
Y
Fig. 10.
If the “curve” happens to be a straight line, like th at in Fig. 12,the
value of
dy
dx
will be the same at all points along i t . In other words its
slope is constant.
If a cur ve is one that turn s mor e u pwards as it goes along to the
right, the values of
dy
dx
will b ecom e greater and greater with th e in-
creasing steepness, as in Fig. 13.
If a curve is one that gets flatter and flatter as it goes along, the
values of
dy
dx
will become smaller and smaller as the flatter par t is
dx
dy
Q
y
x
dx
O X
Y
Fig. 11.
MEANING OF DIFF ER ENTI ATION 79
dx
dy
dx
dy
dx
dy
O X
Y
Fig. 12.
dx
dy
dx
dy
dx
dy
O X
Y
Fig. 13.
reached, as in Fig. 14.
If a curve first descends, and then goes u p agai n , as in Fig. 15,
presenting a concavity upwards, then clearly
dy
dx
will first be negative,
with diminishing values as the curve flattens, then will be zero at the
point where the bottom of the trough of the curve is reached; and from
this point onward
dy
dx
will have positive values that go on incr easin g. In
such a case y is said to pass by a minimum.Theminimumvalueofy is
not necessarily the smallest value of y,itisthatvalueofy corresponding
to the bottom of the trough; for instance, in Fig. 28 (p. 99), the value
of y corr espondi n g to the bottom of the trough is 1, while y takes
CALCULUS MADE EASY 80
O X
Y
Fig. 14.
O X
Y
y min.
Fig. 15.
elsewhere values which are smaller than this. The characteristic of a
minimum is that y must increase on either side of it.
N.B.—For the particular value of x that makes y a minimum,the
value of
dy
dx
=0.
If a curve first ascends and then descends, the values of
dy
dx
will be
positive at first; then zero, as the summit is reached; then negative,
as the curve slopes downwards, as in Fig . 16.Inthiscasey is said to
pass by a maximum,butthemaximumvalueofy is not necessarily the
greatest value of y.InFig. 28,themaximumofy is 2
1
3
,butthisis
by no means the greatest value y can have at some other point of the
curve.
N.B.—For the particular value of x that makes y a maximum,the
value of
dy
dx
=0.
If a curve has the peculiar form of Fig. 17,thevaluesof
dy
dx
will
always be positive; but there will be one particular place where t h e
slope is least steep, where the value of
dy
dx
will be a minimum; that is,
less than it is at any other part of the curve.
MEANING OF DIFF ER ENTI ATION 81
O X
Y
y max.
Fig. 16.
O X
Y
Fig. 17.
If a curve has the form of Fi g . 18,thevalueof
dy
dx
will be negative
in the upper part, and positive in the lower part; while at the nose of
the curve where it becomes actually perpendicular, the value of
dy
dx
will
be infinitely great.
dx
dx
dy
dy
Q
O X
Y
Fig. 18.
Now that we understand that
dy
dx
measures the steepness of a curve
at any point, let us turn to some of the equations which we have already
learned how to di↵erentiate.
CALCULUS MADE EASY 82
(1) As the simplest case take this:
y = x + b.
It is plotted out in Fig. 19,usingequalscalesforx and y.Ifweput
x =0,thenthecorrespondingordinatewillbey = b;thatistosay,the
“curve” crosses the y-axi s at the height b.Fromhereitascendsat45
;
dx
dy
b
O X
Y
Fig. 19.
b
O X
Y
Fig. 20.
for whatever values we give to x to the right, we have an equal y to
ascend. The line has a gradient of 1 in 1.
Now di↵erentiate y = x + b,bytheruleswehavealreadylearned
(pp. 21 and 25 ante), and we get
dy
dx
=1.
The slope of the l i n e is such t h at for every little step dx to the right,
we go an equal little step dy upward. And this slope is constant—always
the same slope.
(2) Take another case:
y = ax + b.
MEANING OF DIFF ER ENTI ATION 83
We know that this curve, like the preceding one, will start from a
height b on the y-axis. But before we draw the curve, let us find i ts
slope by di↵erentiating; which gives
dy
dx
= a.Theslopewillbeconstant,
at an angle, the tangent of which is here called a.Letusassigntoa
some numerical value—say
1
3
. Then we must give it such a slope that
it ascends 1 in 3; or dx will be 3 times as great as dy;asmagnifiedin
Fig. 21.So,drawthelineinFi g. 20 at this slope.
Fig. 21.
(3) Now for a slightly harder case.
Let y = ax
2
+ b.
Again the curve will start on the y-axis at a height b above the
origin.
Now di↵erentiat e. [If you have forgotte n , turn back to p. 25;or,
rather, don’t turn back, but think out the di↵erentiation.]
dy
dx
=2ax.
This shows that the steepness will not be constant: it increases as
x increases. At the starting point P ,wherex =0,thecurve(Fig. 22)
has no steepness—that is, it is level. On the left o f the origin, where x
has negative values,
dy
dx
will also have negative valu es, or will descend
from left to right, as in the Figure.
CALCULUS MADE EASY 84
P
Q
R
b
O X
Y
Fig. 22.
Let us illustrate this by working out a particular instance. Taking
the equation
y =
1
4
x
2
+3,
and di↵erentiating it, we get
dy
dx
=
1
2
x.
Now assign a few successive values, say from 0 to 5, to x;andcalculate
the corresponding values of y by the first equation; and of
dy
dx
from the
second equat i on . Tab u l at i ng results, we have:
x 0 1 2 3 4 5
y 3 3
1
4
4 5
1
4
7 9
1
4
dy
dx
0
1
2
1 1
1
2
2 2
1
2
Then plot them out in two curves, Figs. 23 and 24,inFig. 23 plotting
the values of y against those of x and in Fig. 24 those of
dy
dx
against
those of x.Foranyassignedvalueofx,theheight of the ordinate in
the second curve is proportional to the slope of the first curve.
MEANING OF DIFF ER ENTI ATION 85
3 2 10 1 2 3 4 5
5
6
7
8
9
x
y
b
1
4
x
2
y =
1
4
x
2
+3
Fig. 23.
3 2 1
12345
1
2
3
4
5
x
dy
dx
0
dy
dx
=
1
2
x
Fig. 24.
If a curve comes to a sudden cusp, as in Fig. 25,theslopeatthat
point suddenly changes from a slope upward to a slope downward. In
O X
Y
Fig. 25.
that case
dy
dx
will clearly undergo an abrupt change from a positive to
anegativevalue.
The following examples show further applications of the prin cip l es
just explain ed .
CALCULUS MADE EASY 86
(4) Find the slope of the tangent to the curve
y =
1
2x
+3,
at the point where x = 1. Find the angle which this tangent makes
with the curve y =2x
2
+2.
The slope of the tangent is the slope of t h e curve at the point where
they touch one another (see p. 76); that is, it is the
dy
dx
of the curve for
that point. Here
dy
dx
=
1
2x
2
and for x = 1,
dy
dx
=
1
2
,whichisthe
slope of the tangent and of the curve at that point. The tangent, being
astraightline,hasforequationy = ax + b,anditsslopeis
dy
dx
= a,
hence a =
1
2
. Also if x = 1, y =
1
2(1)
+3 = 2
1
2
;andasthe
tangent passes by this point, the coordinates of the point must satisfy
the equation of the tangent, namely
y =
1
2
x + b,
so that 2
1
2
=
1
2
⇥ (1) + b and b = 2; the eq u a t i on of the tangent is
therefore y =
1
2
x +2.
Now, when two curves meet, the intersection being a point com-
mon to both curves, its co ordinates must satisfy the equation of each
one of the two curves; that is, it must be a solution of the system of
simultaneous equations formed by coupling together the equations of
the curves. Here the curves meet o n e another at points given by the
solution of
8
<
:
y =2x
2
+2,
y =
1
2
x +2 or 2x
2
+2=
1
2
x +2;
MEANING OF DIFF ER ENTI ATION 87
that is, x(2x +
1
2
)=0.
This equation has for its solutions x =0andx =
1
4
.Theslopeof
the curve y =2x
2
+2atanypointis
dy
dx
=4x.
For the point where x = 0, this slope is zero; the curve is h or i zo ntal.
For the p oint where
x =
1
4
,
dy
dx
= 1;
hence the curve at that point slopes downwards to the right at such
an angle ✓ with the horizontal that tan ✓ = 1; that is, at 45
to the
horizontal.
The slope of the straight line is
1
2
;thatis,itslopesdownwardsto
the right and makes with the horizontal an angle such that t an =
1
2
;
that is, an angle of 26
34
0
.Itfollowsthatatthefirstpointthecurve
cuts the straight line at an angle of 26
34
0
,whileattheseconditcuts
it at an angle of 45
26
34
0
=18
26
0
.
(5) A straight line is to be drawn, through a point whose coordinates
are x =2,y = 1, as tangent to the curve y = x
2
5x + 6. Find the
coordinates of the p oi nt of contact.
The sl ope of the tangent must be the same as the
dy
dx
of the curve;
that is, 2x 5.
The equation of the straight line is y = ax+b,andasitissatisfiedfor
the values x =2,y = 1, then 1=a⇥2+b;also,its
dy
dx
= a =2x5.
The x and the y of the point of contact must also satisfy both the
equation o f the tangent and the equation of the curve.
CALCULUS MADE EASY 88
We have then
y = x
2
5x +6, (i)
y = ax + b, (ii)
8
>
>
>
>
>
>
<
>
>
>
>
>
>
:
1=2a + b, (iii)
a =2x 5, (iv)
four equation s in a, b, x, y.
Equations (i ) and (ii) give x
2
5x +6=ax + b.
Replacing a and b by their v alue in this, we get
x
2
5x +6=(2x 5)x 1 2(2x 5),
which simpli fi es to x
2
4x +3=0,thesolutionsofwhich are: x =3
and x =1. Replacingin(i),wegety =0andy =2respectively;the
two points of contact are then x =1,y =2,andx =3,y =0.
Note.—In all exercises dealing with curves, students will find it ex-
tremely instructive to verify the deductions obtained by actually plot-
ting the curves.
Exercises VII I . (See page 256 for Answers.)
(1) Plot the c urve y =
3
4
x
2
5, using a scale of millimetres. Measure
at points corresponding to di↵erent values of x,theangleofitsslope.
Find, by di↵erentiating the equation, the expression for slope; and
see, from a Table of Natural Tangents, whether this agrees with the
measured angle.
MEANING OF DIFF ER ENTI ATION 89
(2) Find what will be the slope of the curve
y =0.12x
3
2,
at the particular point that has as abscissa x =2.
(3) If y =(x a)(x b) , show that at the particular point of the
curve wh er e
dy
dx
=0,x will have the value
1
2
(a + b).
(4) Find the
dy
dx
of the equation y = x
3
+3x;andcalculatethe
numerical values of
dy
dx
for the points corresponding to x =0,x =
1
2
,
x =1,x =2.
(5) In the curve to which the equation is x
2
+y
2
=4,findthevalues
of x at those points where the slope = 1.
(6) Find the slope, at any point, of the curve whose equation is
x
2
3
2
+
y
2
2
2
=1;andgivethenumericalvalueoftheslopeattheplace
where x =0,andatthatwherex =1.
(7) The equation of a tangent to the curve y =52x +0.5x
3
,being
of the form y = mx + n,wherem and n are constants , find the value
of m and n if the point where the tangent touches the curve has x =2
for abscissa.
(8) At what angle do the two curves
y =3.5x
2
+2 and y = x
2
5x +9.5
cut one another?
(9) Tangents to the curve y = ±
p
25 x
2
are drawn at points for
which x =3andx = 4. Fin d the coordinates of the point of intersection
of the tangents and their mutual inclination.
CALCULUS MADE EASY 90
(10) Astraightliney =2x b touches a curve y =3x
2
+2at one
point. What are the coordinat es of the point of contact, and what is
the value of b?
The simplest case to examine is that of a linear function. The slope $a$ of a linear function is a constant. In this first example the author choose the easiest of all $a=1$.
This is a great example where you can visualize the graph for a given function $y=f(x)$ on Figure 23 and the graph for it's derivative $\frac{dy}{dx}=f^{\prime}(x)$ of that function Figure 24.
local minimum
The tangent to the curve at specific point is parametrized by a linear function as follows $y = ax+b$ where a is the slope of that curve and is computed as follows:
\begin{eqnarray*}
a&=&{\frac {{\text{change in }}y}{{\text{change in }}x}}\\
&=&{\frac {\Delta y}{\Delta x}}\\
&=&{\tan{\theta}}
\end{eqnarray*}

*Figure: Slope of a linear function.*
Values for the tangent function $\tan$ between $-2\pi$ and $2\pi$. Note that for an angle $\theta = 45^{\circ} = \frac{\pi}{4}$:
$\tan{(45^{\circ})}= \tan{(\frac{\pi}{4})} = 1$

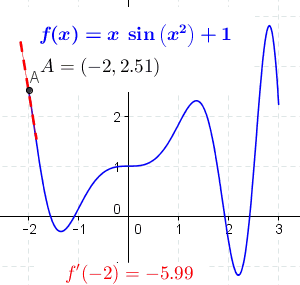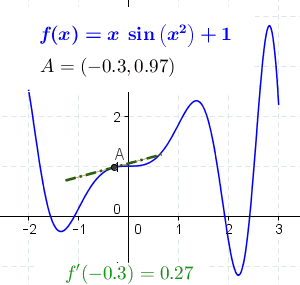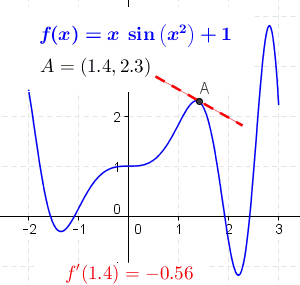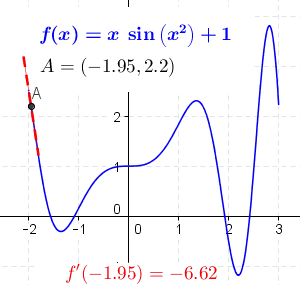
A visual example of varying slopes at different points of a curve.
Here the values for the slope are given by $f^{\prime}$ at different points $A$ for the function $f(x)=x\sin{(x^2)}+1$.
let's talk about this
ahhhh
That's funny
In this figure we can see one maximum at $x=1$ and one minimum at $x=3$. The slope **a** of the tangent to the curve at those points is 0, i.e. $a=\frac{dy}{dx} = 0$. To the left of a minimum we have that $a<0$ and to the right $a>0$. Inversely for a maximum we have that to the left of it the slope is $a>0$ and to the right $a<0$.

Silvanus Phillips Thompson was a professor of physics mostly known for his work as an electrical engineer. He was elected to the Royal Society 1891. Thompson was also an author and he published his most famous book **Calculus Made Easy** in 1910. This book is one of the greatest introductions to the fundamentals of infinitesimal calculus, and is still in print today.
Learn more about the author here:
- [Silvanus P. Thompson 1851-1916
Professor of Physics - Electrical Engineer](http://www.engineerswalk.co.uk/st_walk.html)
- [Silvanus P. Thompson](https://en.wikipedia.org/wiki/Silvanus_P._Thompson)

You can find the entire book here:
- [Calculus Made Easy (HTML version)](https://calculusmadeeasy.org)
- [The Project Gutenberg EBook of Calculus Made Easy, by Silvanus Thompson (PDF version)](http://www.gutenberg.org/files/33283/33283-pdf.pdf)
### TL;DR
This is a text about differentiation and its geometrical meaning from the book "Calculus Made Easy" originally published in 1910 by Silvanus P. Thompson. It constitutes one of the greatest and most elegant introductions to the topic of calculus. It's worth reading even if you know math really well because it will help you understand how to teach complexity without any added fluff or overt technicality to others. It's a fascinating book.
Excerpt from its Prologue:
> ***Considering how many fools can calculate, it is surprising that it should be thought either a difficult or a tedious task for any other fool to learn how to master the same tricks.***
>*** Some calculus tricks are quite easy. Some are enormously difficult. The fools who write the textbooks of advanced mathematics - and they are mostly clever fools - seldom take the trouble to show you how easy the easy calculations are. On the contrary, they seem to desire to impress you with their tremendous cleverness by going about it in the most difficult way.***
>*** Being myself a remarkably stupid fellow, I have had to unteach myself the difficulties, and now beg to present to my fellow fools the parts that are not hard. Master these thoroughly, and the rest will follow. What one fool can do, another can.***